refer to scenario 1. what is the idle time per cycle at ws 4?
Mathematics (maths) - Queueing Theory - Important Brusk Objective Questions and Answers: Queueing Theory
Queueing Theory
1) What is meant past queue Discipline ?
Answer:
Information technology Specifies the manner in which the customers from the queue or equivalently the manner in which they are selected for service, when a queue has been formed. The most mutual discipline are
(i) FCFS (First Come up Beginning Served) or First In Commencement Out (FIFO)
(ii) LCFS (Last Come First Served)
(3) SIRO (Service in Random Order)
2) Define Little's formula

three) If people go far to buy cinema tickets at the boilerplate rate of vi per infinitesimal, it takes an boilerplate of vii.5 seconds to purchase a ticket. If a person arrives 2 minutes before the film starts and if it takes exactly one.5 minutes to reach the correct seat subsequently purchasing the ticket. Can he look to be seated for the start of the picture ?
Answer:

4) If λ , µ are the rates of arrival and departure in a M/Chiliad/I queue respectively, give the formula for the probability that there are n customers in the queue at any fourth dimension in steady state.
Answer:

5) If λ , µ are the rates of inflow and divergence respectively in a M/M/I queue, write the formulas for the average waiting time of a customer in the queue and the average number of customers in the queue in the steady state.
Answer:
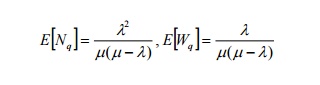
6) If the inflow and deviation rates in a public phone booth with a unmarried phone are
1/12 and1 /14 respectively, observe the probability that the phone is decorated.
Reply:
P[Phone is busy] = 1 – P [ No customer in the booth]

vii) If the inter-arrival time and service fourth dimension in a public phone booth with a unmarried-phone follow exponential distributions with ways of 10 and viii minutes respectively, Discover the average number of callers in the berth at any fourth dimension.
Reply:
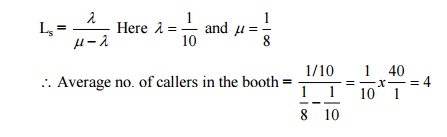
8) If the inflow and departure rates in a M/G/I queue are 1/ii per minute and 2/3 per minute respectively, detect the average waiting time of a customer in the queue.
Answer:
Average waiting fourth dimension of a customer in the queue
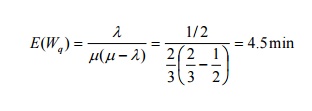
9) Customers make it at a railway ticket counter at the charge per unit of 30/hour. Bold Poisson arrivals, exponential service time distribution and a single server queue (Yard/G/I) model, find the boilerplate waiting time (before being served) if the average service time is 100 seconds.
Reply:
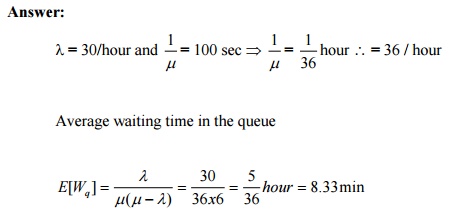
10) What is the probability that a customer has to wait more than 15 minutes to become his service completed in (M/Grand/I) : ( ∞ / FIFO) queue systme if λ = 6 per 60 minutes and µ = x per hour ?
Respond:
probability that the waiting fourth dimension of a customer in the arrangement exceeds time t=due east − ( µ − λ )t

11) For (M/Thousand/I) : ( ∞ / FIFO) model, write down the Picayune's formula.
Answer:
(i) E(Norths) = λ Due east(Ws)
(ii) (ii) Eastward(Nq) = λ East(Wq)

12) Using the Trivial's formula, obtain the average waiting fourth dimension in the system for M|M|1|N model.
Answer:
By the modified Piffling's formula,
Eastward(WS) = 1/ λ′ East (NS) where λ′ is the constructive arrival rate.
thirteen) For (G/Grand/I) : ( ∞ / FIFO) model, write downward the formula fora.Boilerplate number of customers in the queue
b. Average waiting time in the arrangement.
Answer:

14) What is the probability that a customer has to wait more than than xv minutes to become his service completed in Thousand|1000|ane queuing system, if λ = vi per hour and µ = x per hour ? Respond:
Probability that the waiting fourth dimension in the system exceeds t is

15) What is the probability that an arrival to an infinite capacity three server Poisson queuing

P [with out waiting] = P [Northward < iii ] = P0 + P1 + P2
16) Consider an M|Grand|1 queuing system. If λ = six and µ = viii, Find the probability of atleast 10 customers in the system.
Answer:
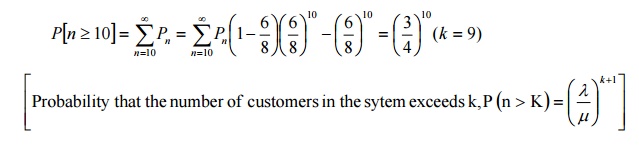
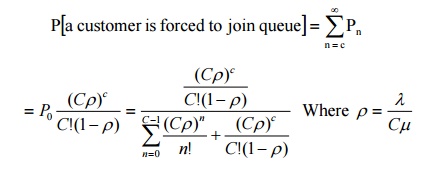
17) Consider an M|1000|C queuing organization. Observe the probability that an arriving customer is forced to join the queue.
Answer:
eighteen) Write down Pollaczek-Khinchine formulae.

nineteen) Consider an M|M|1 queueing system. Discover the probability of finding atleast 'n' customers in the system.
Respond:
Probability of at least n customers in the system
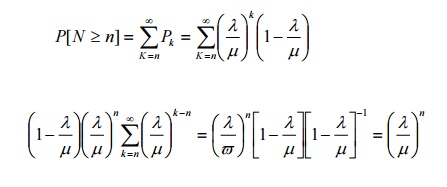
xx) Consider an M|M|C queueing organization. Notice the probability that an arriving customer is forced to bring together the queue.
Answer:
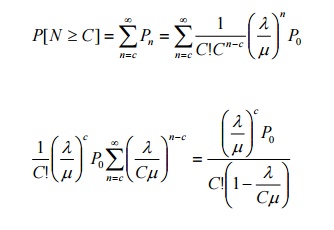
21.Briefly draw the M|G|1 queuing arrangement. Reply:
Answer:
Poisson arrival / Full general service / Single server queuing system.
22) Arrivals at a telephone booth are considered to be Poisson with an average time of 12 minutes between 1 inflow and the side by side. The length of a telephone call is assumed to be exponentially distributed with hateful four min. What is the probability that information technology will take him more than x minutes altogether to look for the phone and consummate his call ?
Answer:
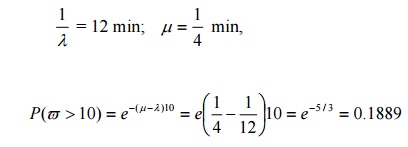
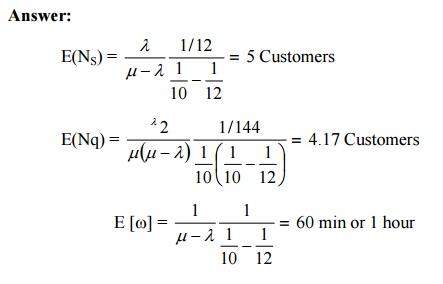
23) Customers arrive at a one-man barber store according to a Poisson process with hateful inter-arrival time of 12 infinitesimal, Customers spend an boilerplate of ten min in the hairdresser's chair. What is the expected number of customers in the barber shop and in the quene ? How much fourth dimension tin can a customer await to spend in the barber's shop ?
Answer:
24) A duplication auto maintained for office apply is operated by office banana. The time to complete each job varies according to an exponential distribution with mean 6 min. Assume a Poisson input with an average inflow charge per unit of v jobs per hour. If an 8-hr day is used as a base, determine
a) The per centum of idle time of the machine.
b) The boilerplate time a chore is in the arrangement.
Respond:

25 ) In a (M|1000|1):( ∞ /F1F0) queuing model, the inflow and service rates are λ = 12/ hour
and µ = 24/hour, discover the average number of customers in the system and in the queue.
Answer:
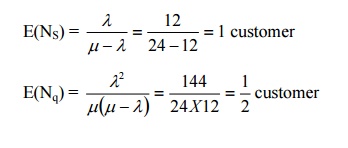
26) Customers arrive at a one-man barber shop according to a Poisson process with a hateful inter arrival time of 12 minute. Customers spend an average of 10 minutes in the hairdresser'south chair, what is the probability that more than than 3 customers are in the organisation ?
Answer:
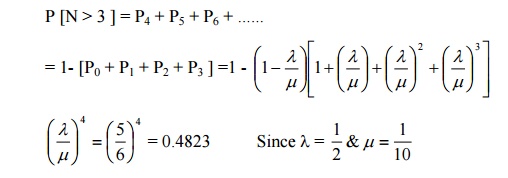
27) If a customer has to wait in a (1000|M|1):( ∞ /F1F0) queue arrangement what is his average waiting time in the queue, if λ = viii per hour and µ =12 per 60 minutes ?
Answer:
Average waiting time of a customer in the queue, if he has to wait.
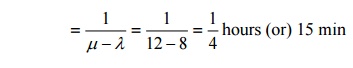
28) What is the probability that a customer has to wait more than 15 minutes to become his service completed in (M|M|1):( ∞ /F1F0) queue arrangement, if λ = half-dozen per hour and µ = 10 per hour. Answer:

29) If there are two servers in an infinite capacity Poisson queue system with λ =10 and µ =15 per hour, what is the per centum of idle time for each server ?
Answer:
P [ the server will be idle] = P0
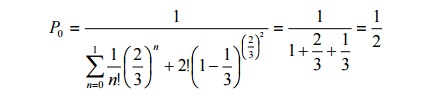
Pct of idle time for each server = l%
30) If λ = 4 per hr and µ = 12 per 60 minutes in an (M|Chiliad|i):( ∞ /F1F0) queuing system, find the probability that there is no customer in the organization.
Answer:
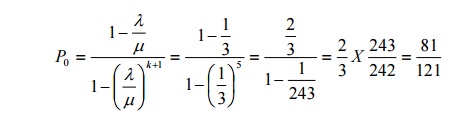
Study Material, Lecturing Notes, Consignment, Reference, Wiki description explanation, brief particular
Mathematics (maths) : Queueing Theory : Important Short Objective Questions and Answers: Queueing Theory |
Source: https://www.brainkart.com/article/Important-Short-Objective-Questions-and-Answers--Queueing-Theory_6481/

0 Response to "refer to scenario 1. what is the idle time per cycle at ws 4?"
Post a Comment